🪴 Anil's Garden

Selection Sort Algorithm - GeeksforGeeks

Excerpt

Selection sort is a simple and efficient sorting algorithm that works by repeatedly selecting the smallest (or largest) element from the unsorted portion of the list and moving it to the sorted portion of the list. The algorithm repeatedly selects the smallest (or largest) element from the unsorted portion of

Last Updated : 06 Aug, 2024

Selection sort is a simple and efficient sorting algorithm that works by repeatedly selecting the smallest (or largest) element from the unsorted portion of the list and moving it to the sorted portion of the list.

The algorithm repeatedly selects the smallest (or largest) element from the unsorted portion of the list and swaps it with the first element of the unsorted part. This process is repeated for the remaining unsorted portion until the entire list is sorted.

How does Selection Sort Algorithm work?

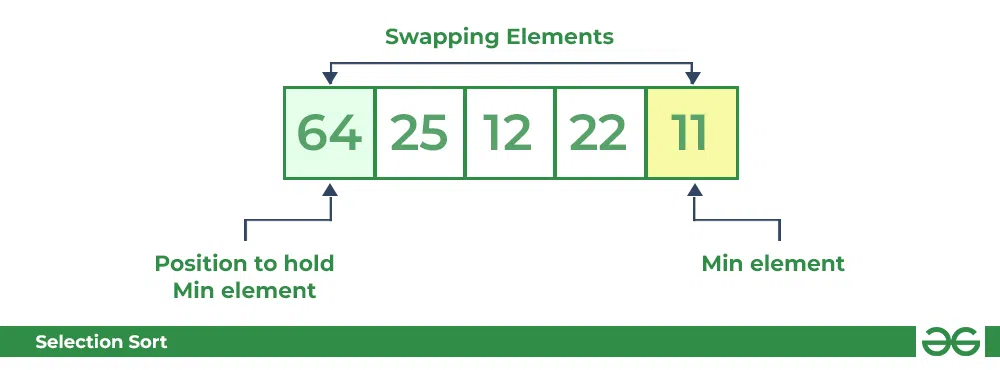
Lets consider the following array as an example: arr[] = {64, 25, 12, 22, 11}

First pass:

For the first position in the sorted array, the whole array is traversed from index 0 to 4 sequentially. The first position where 64 is stored presently, after traversing whole array it is clear that 11 is the lowest value.

Thus, replace 64 with 11. After one iteration 11, which happens to be the least value in the array, tends to appear in the first position of the sorted list.

Selection Sort Algorithm | Swapping 1st element with the minimum in array

Second Pass:

For the second position, where 25 is present, again traverse the rest of the array in a sequential manner.

After traversing, we found that 12 is the second lowest value in the array and it should appear at the second place in the array, thus swap these values.

Selection Sort Algorithm | swapping i=1 with the next minimum element

Third Pass:

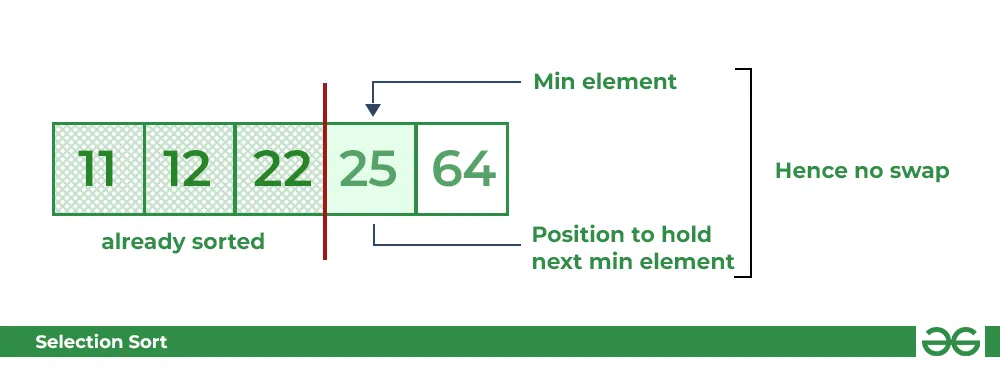
Now, for third place, where 25 is present again traverse the rest of the array and find the third least value present in the array.

While traversing, 22 came out to be the third least value and it should appear at the third place in the array, thus swap 22 with element present at third position.

Selection Sort Algorithm | swapping i=2 with the next minimum element

Fourth pass:

Similarly, for fourth position traverse the rest of the array and find the fourth least element in the array

As 25 is the 4th lowest value hence, it will place at the fourth position.

Selection Sort Algorithm | swapping i=3 with the next minimum element

Fifth Pass:

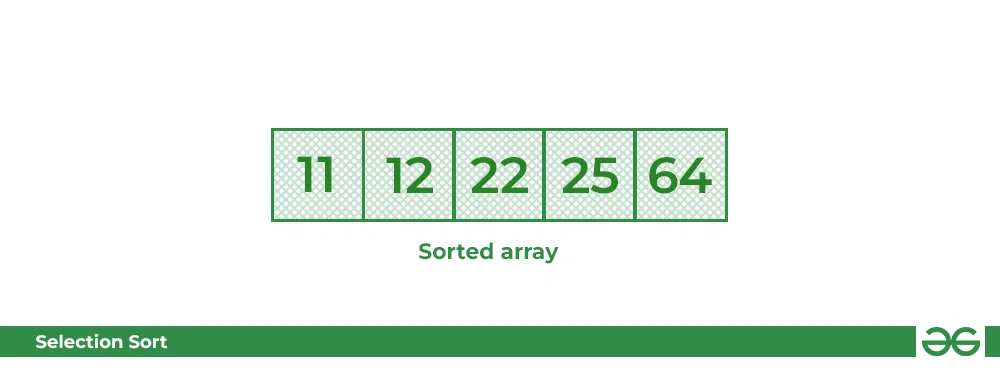
At last the largest value present in the array automatically get placed at the last position in the array

The resulted array is the sorted array.

Selection Sort Algorithm | Required sorted array

Below is the implementation of the above approach:

C++ C Java Python``` # Python program for implementation of Selection # Sort A = [64, 25, 12, 22, 11] # Traverse through all array elements for i in range(len(A)-1): # Find the minimum element in remaining # unsorted array min_idx = i for j in range(i+1, len(A)): if A[min_idx] > A[j]: min_idx = j # Swap the found minimum element with # the first element A[i], A[min_idx] = A[min_idx], A[i] # Driver code to test above print ("Sorted array") for i in range(len(A)): print(A[i],end=" ") ``` C# JavaScript PHP

Output

Sorted array: 
11 12 22 25 64

Complexity Analysis of Selection Sort

Time Complexity: The time complexity of Selection Sort is O(N**²****)** as there are two nested loops:

One loop to select an element of Array one by one = O(N)
Another loop to compare that element with every other Array element = O(N)
Therefore overall complexity = O(N) * O(N) = O(N*N) = O(N²)

Auxiliary Space: O(1) as the only extra memory used is for temporary variables while swapping two values in Array. The selection sort never makes more than O(N) swaps and can be useful when memory writing is costly.

Advantages of Selection Sort Algorithm

Simple and easy to understand.
Works well with small datasets.

Disadvantages of the Selection Sort Algorithm

Selection sort has a time complexity of O(n^2) in the worst and average case.
Does not work well on large datasets.
Does not preserve the relative order of items with equal keys which means it is not stable.

Applications of Selection Sort Algorithm

Mainly works as a basis for some more efficient algorithms like Heap Sort. Heap Sort mainly uses Heap Data Structure along with the Selection Sort idea.
Used when memory writes (or swaps) are costly for example EEPROM or Flash Memory. When compared to other popular sorting algorithms, it takes relatively less memory writes (or less swaps) for sorting. But Selection sort is not optimal in terms of memory writes, cycle sort even requires lesser memory writes than selection sort.
Simple technique and used to introduce sorting in teaching.
Used as a benchmark for comparison with other algorithms.

Frequently Asked Questions on Selection Sort

Q1. Is Selection Sort Algorithm stable?

The default implementation of the Selection Sort Algorithm is not stable. However, it can be made stable. Please see the stable Selection Sort for details.

Q2. Is Selection Sort Algorithm in-place?

Yes, Selection Sort Algorithm is an in-place algorithm, as it does not require extra space.

Are you looking to bridge the gap from Data Structures and Algorithms (DSA) to Software Development? Dive into our DSA to Development - Beginner to Advance Course on GeeksforGeeks, crafted for aspiring developers and seasoned programmers alike. Explore essential coding skills, software engineering principles, and practical application techniques through hands-on projects and real-world examples. Whether you’re starting your journey or aiming to refine your skills, this course empowers you to build robust software solutions. Ready to advance your programming prowess? Enroll now and transform your coding capabilities!

🪴 Anil's Garden

Explorer

Selection Sort Algorithm - GeeksforGeeks

Selection Sort Algorithm - GeeksforGeeks

Excerpt

How does Selection Sort Algorithm work?

Complexity Analysis of Selection Sort

Advantages of Selection Sort Algorithm

Disadvantages of the Selection Sort Algorithm

Applications of Selection Sort Algorithm

Frequently Asked Questions on Selection Sort

Graph View

Table of Contents

Backlinks